What Is The Multiplicative Rate Of Change Of The Function?

Understanding the Multiplicative Rate of Change of a Function

The concept of the multiplicative rate of change is a fundamental aspect of mathematics, particularly in the study of functions and their behaviors. It plays a crucial role in various fields, including economics, biology, physics, and finance. This article will provide a comprehensive overview of the multiplicative rate of change, how to calculate it, its applications, and examples to illustrate the concept.

What is the Multiplicative Rate of Change?

Definition

The multiplicative rate of change refers to the factor by which a quantity changes relative to its previous value. It is often expressed as a percentage or a decimal and is particularly relevant in the context of exponential functions, where the rate of change is proportional to the current value.

Mathematical Representation

The multiplicative rate of change can be mathematically represented as:

Where:

• f(x1)f(x1​) is the initial value of the function at point x1x1​.
• f(x2)f(x2​) is the value of the function at point x2x2​.

Alternatively, it can also be expressed as:

This formula provides a clear understanding of how much a function has increased or decreased relative to its previous value.

Characteristics of Multiplicative Rate of Change

1. Exponential Growth and Decay

The multiplicative rate of change is particularly significant in exponential functions, where it determines how quickly a quantity grows or decays over time. For example, in a population growth model, the multiplicative rate of change indicates how much the population increases each year.

2. Constant vs. Variable Rates

• Constant Rate: In some functions, the multiplicative rate of change remains constant over time. This means that the function grows or decays by the same percentage each time period.
• Variable Rate: In other cases, the multiplicative rate of change can vary, leading to more complex behaviors in the function. This is often seen in real-world scenarios where external factors influence growth or decay.

3. Application in Real Life

Understanding the multiplicative rate of change is essential for analyzing various phenomena, including:

• Finance: Calculating interest rates, investment growth, and inflation.
• Biology: Studying population dynamics and the spread of diseases.
• Physics: Analyzing decay rates of radioactive substances.

How to Calculate the Multiplicative Rate of Change

Example 1: Exponential Growth

Consider a population of bacteria that doubles every hour. If the initial population is 100, we can calculate the multiplicative rate of change after one hour.

1. Initial Value: f(x1)=100f(x1​)=100
2. Value After 1 Hour: f(x2)=200f(x2​)=200

Using the formula:

This indicates a multiplicative rate of change of 1, or a 100% increase in the population.

Example 2: Exponential Decay

Now consider a radioactive substance that decays by 25% every hour. If the initial amount is 80 grams, we can calculate the multiplicative rate of change after one hour.

1. Initial Value: f(x1)=80f(x1​)=80
2. Value After 1 Hour: f(x2)=80−(0.25×80)=60f(x2​)=80−(0.25×80)=60

Using the formula:

This indicates a multiplicative rate of change of -0.25, or a 25% decrease in the amount of the substance.

Applications of Multiplicative Rate of Change

1. Financial Analysis

In finance, the multiplicative rate of change is used to calculate the growth of investments, interest rates, and returns on investment. For example, if an investment grows from $1,000 to $1,200 over a year, the multiplicative rate of change can be calculated as follows:

This indicates a 20% increase in the investment.

2. Population Studies

In biology, the multiplicative rate of change helps researchers understand population dynamics. For example, if a population of rabbits increases from 200 to 300 in a year, the multiplicative rate of change is:

This indicates a 50% increase in the rabbit population.

3. Environmental Science

In environmental studies, the multiplicative rate of change can be used to analyze the spread of invasive species or the recovery of endangered species. For example, if an endangered species population grows from 50 to 75 individuals, the multiplicative rate of change is:

This indicates a 50% increase in the endangered species population.

Factors Affecting the Multiplicative Rate of Change

1. Initial Conditions

The initial value of a function can significantly impact the multiplicative rate of change. For example, a larger initial value may lead to a higher absolute increase, even if the percentage increase remains constant.

2. External Influences

External factors, such as economic conditions, environmental changes, and social dynamics, can influence the rate of change. For example, a sudden economic downturn may slow the growth of a business, affecting its multiplicative rate of change.

3. Time Frame

The time frame over which the change occurs can also affect the multiplicative rate of change. Shorter time frames may exhibit more volatility, while longer time frames may show more stable trends.

Understanding Exponential Functions

Definition of Exponential Functions

An exponential function is a mathematical function of the form:

Where:

• $a$ is the initial value (y-intercept).
• $b$ is the base of the exponential function (growth factor if $b>1$ or decay factor if $0<b<1$).
• $x$ is the exponent.

Characteristics of Exponential Functions

1. Rapid Growth or Decay: Exponential functions can grow or decay rapidly, leading to significant changes in value over time.
2. Horizontal Asymptote: Exponential functions have a horizontal asymptote, which is the line that the graph approaches but never touches. For exponential decay, this is typically the x-axis (y=0).
3. Continuous Growth: Exponential functions model continuous growth or decay, making them ideal for representing real-world phenomena.

Example of an Exponential Function

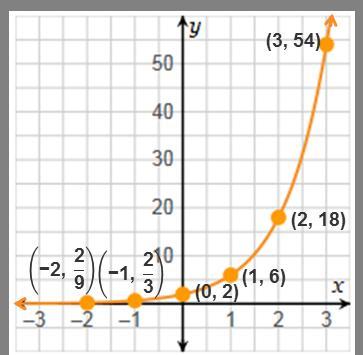
Consider the function f(x)=2⋅3xf(x)=2⋅3x. This function represents exponential growth, where:

• The initial value is 2 (when $x=0$).
• The base is 3, indicating that the function grows by a factor of 3 for each unit increase in $x$.

To find the multiplicative rate of change between $x=1$ and $x=2$:

1. Calculate $f(1)$ and $f(2)$:
   • f(1)=2⋅31=6f(1)=2⋅31=6
   • f(2)=2⋅32=18f(2)=2⋅32=18
2. Use the multiplicative rate of change formula:
   Multiplicative Rate of Change=f(2)f(1)−1=186−1=2Multiplicative Rate of Change=f(1)f(2)​−1=618​−1=2

This indicates a multiplicative rate of change of 2, or a 200% increase.

Conclusion

The multiplicative rate of change is a vital concept in mathematics that helps us understand how functions behave, particularly in exponential growth and decay scenarios. By grasping how to calculate and interpret the multiplicative rate of change, individuals can gain valuable insights into various fields, including finance, biology, and environmental science.Understanding this concept allows for better decision-making, whether you’re analyzing investments, studying population dynamics, or exploring the effects of external influences on growth rates. By applying the knowledge gained in this article, you can navigate the complexities of exponential functions and their real-world applications effectively.

FAQ Section

What is the multiplicative rate of change?

The multiplicative rate of change refers to the factor by which a quantity changes relative to its previous value, often expressed as a percentage or decimal.

How do you calculate the multiplicative rate of change?

To calculate the multiplicative rate of change, use the formula:

Where f(x1)f(x1​) is the initial value and f(x2)f(x2​) is the value after the change.

What is an example of multiplicative rate of change in real life?

An example of the multiplicative rate of change in real life is the growth of a bank investment. If an investment grows from $1,000 to $1,200 in a year, the multiplicative rate of change would be:

What is the difference between multiplicative and additive rate of change?

The multiplicative rate of change refers to the percentage change relative to the previous value, whereas the additive rate of change refers to a constant amount added to the previous value.

Where can I find more information about the multiplicative rate of change?

For more detailed information about the multiplicative rate of change and related mathematical concepts, you can visit the Wikipedia page on Rate of Change. This comprehensive guide provides a detailed understanding of the multiplicative rate of change, its calculations, and its applications.