How To Divide Fractions

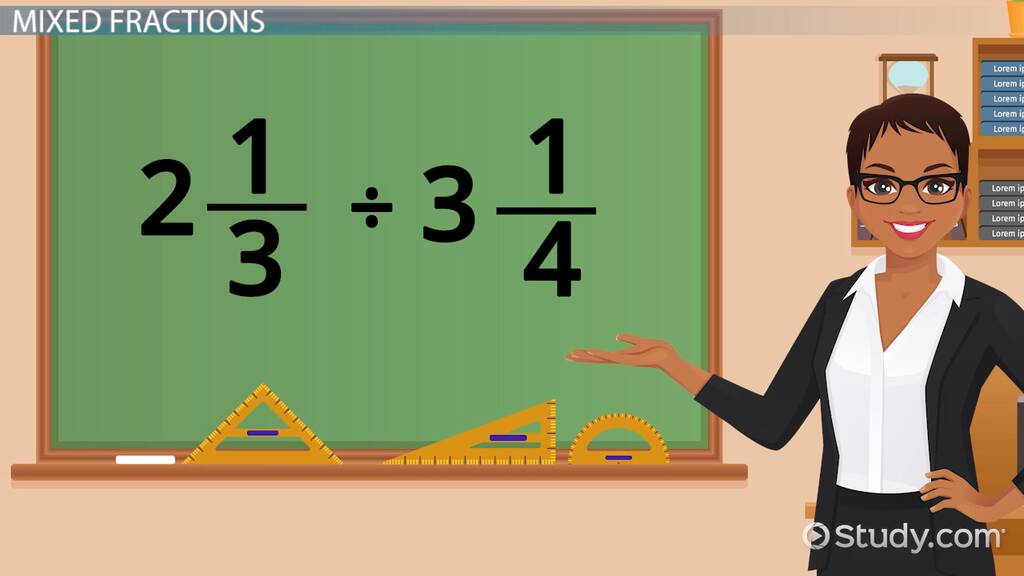
Conquering Fractions: Unveiling the Secrets of Division

Fractions, those numerical expressions representing parts of a whole, can sometimes pose challenges, especially when it comes to division. But fear not! This comprehensive guide empowers you to conquer the task of dividing fractions, equipping you with step-by-step explanations, practical examples, and valuable tips to solidify your understanding.

Demystifying the Challenge: Why Dividing by Fractions Can Be Tricky

Division itself can be conceptually challenging for some. When fractions enter the equation, the process might seem even more daunting. The key to mastering division of fractions lies in understanding a simple yet powerful concept: dividing by a fraction is the same as multiplying by the reciprocal of that fraction.

• Reciprocal: The reciprocal of a fraction is essentially its inverse or “flip.” For example, the reciprocal of ½ (written as 1/2) is 2 (written as 2/1). Multiplying a number by its reciprocal always results in 1.

The Art of Conquering Fractions: A Step-by-Step Guide

Here’s a breakdown of the process for dividing fractions, incorporating the reciprocal concept:

1. Identify the Dividend and Divisor: In a division problem, the number being divided is called the dividend. The number dividing it is called the divisor. In the case of fractions, both the dividend and divisor will be expressed as fractions.

2. Find the Reciprocal of the Divisor: This is the crucial step. Remember, we’re essentially converting the division problem into a multiplication problem. To achieve this, find the reciprocal of the divisor fraction. As mentioned earlier, the reciprocal is obtained by flipping the numerator and denominator of the divisor fraction.

3. Multiply the Dividend by the Reciprocal: Once you have the reciprocal of the divisor, simply multiply the dividend (the first fraction) by this reciprocal. Multiplication of fractions follows a straightforward rule: multiply the numerators and multiply the denominators.

4. Simplify the Result (Optional): The result of your multiplication might be a fraction in its lowest terms. However, there might be room for simplification. Look for common factors (numbers that divide evenly) between the numerator and denominator of the resulting fraction. Dividing both the numerator and denominator by the greatest common factor will simplify the fraction.

Examples to Illuminate the Process: Let’s Practice!

Here are some examples to illustrate the concept of dividing fractions using the reciprocal method:

• Example 1: Dividing ½ by ¼:

  • Step 1: Dividend = ½ (1/2), Divisor = ¼ (1/4)
  • Step 2: Reciprocal of Divisor (¼) = 4/1
  • Step 3: ½ (1/2) x 4/1 = (1/2) x 4 / (2 x 1) = 4/2
  • Step 4 (Optional): Simplify 4/2 by dividing both numerator and denominator by 2. The result is 2.

• Example 2: Dividing ¾ by ⅛:

  • Step 1: Dividend = ¾ (3/4), Divisor = ⅛ (1/8)
  • Step 2: Reciprocal of Divisor (⅛) = 8/1
  • Step 3: ¾ (3/4) x 8/1 = (3 x 8) / (4 x 1) = 24/4
  • Step 4 (Optional): Simplify 24/4 by dividing both numerator and denominator by 4. The result is 6.

By working through these examples, you can gain practical experience with the reciprocal method for dividing fractions.

Beyond the Basics: Strengthening Your Skills

Here are some additional tips to solidify your understanding of dividing fractions:

• Visualize with Fractions Bars or Drawings: If you find the concept abstract, try using fraction bars or diagrams to represent the fractions and visualize the division process.

• Practice Makes Perfect: The more you practice dividing fractions, the more comfortable you’ll become. Look for practice problems in textbooks, online resources, or create your own!

• Don’t Be Afraid to Simplify as You Go: While the final step encourages simplification, you can also simplify fractions as you multiply during step 3. This can sometimes make the calculations easier to handle.

FAQ: Unveiling the Mysteries of Fraction Division

This section addresses frequently asked questions regarding dividing fractions:

• Is there another way to divide fractions besides the reciprocal method?

There are alternative methods for dividing fractions but the reciprocal method is generally considered the simplest and most efficient approach. Here’s a brief mention of another method, though it might involve more steps:

• Invert the Divisor and Multiply: This method involves flipping the divisor (like the reciprocal method) but without explicitly calculating the reciprocal. Instead of finding the reciprocal (which is essentially flipping the divisor), you directly flip the divisor and then perform the multiplication as usual.

However, for most purposes, the reciprocal method remains the preferred approach due to its simplicity.

• What if I get stuck on a specific problem?

Don’t hesitate to break down the problem step-by-step. Write down each step explicitly (identifying dividend and divisor, finding the reciprocal, multiplying, and simplifying). If you’re still stuck, consult a math tutor, textbook resources, or online tutorials for additional guidance.

• Are there any real-world applications of dividing fractions?

Absolutely! Dividing fractions has practical applications in various fields. For example:

• Cooking: When scaling down a recipe, you might need to divide recipe quantities (which are often fractions) by a specific number of servings.
• Construction: Dividing lengths or areas (expressed as fractions) can be necessary for carpentry projects or calculating materials needed.
• Science: Scientific formulas often involve calculations with fractions, and division of fractions might be necessary within those calculations.

By mastering the concept of dividing fractions, you equip yourself with a valuable mathematical skill applicable in various contexts beyond classroom exercises.

With a solid understanding of the reciprocal method, practice, and these valuable tips, you’ll be well on your way to conquering the world of fractions and confidently tackling division problems!