Conquering the Square: Mastering the Art of Completing the Square
The concept of completing the square might seem daunting at first glance. But fear not, math adventurers! This comprehensive guide will equip you with the knowledge and practice to tackle this technique with confidence.
We’ll delve into the purpose of completing the square, explore the step-by-step process, and apply it to various scenarios. Buckle up and get ready to unlock the secrets of this powerful mathematical tool!
Why Complete the Square? Unveiling the Purpose
Completing the square serves several crucial functions in algebra:
- Factoring Quadratic Expressions: This technique allows you to transform a quadratic expression (ax^2 + bx + c) into a perfect square trinomial, making it easier to factor.
- Solving Quadratic Equations: By completing the square, you can manipulate a quadratic equation (ax^2 + bx + c = 0) into a form that reveals the solutions (roots) of the equation.
- Understanding the Vertex Form: Completing the square helps visualize the vertex form of a quadratic equation, which provides valuable insights into the graph’s behavior (axis of symmetry, minimum or maximum point).
In essence, completing the square unlocks a pathway to solving various quadratic problems and gaining a deeper understanding of their characteristics.
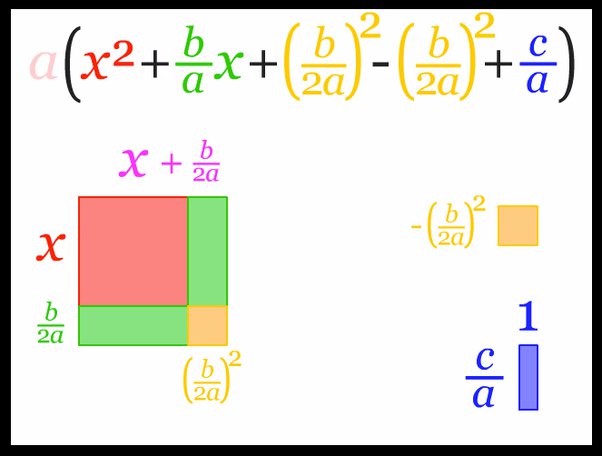
The Formula Unveiled: A Step-by-Step Guide
The process of completing the square involves manipulating a quadratic expression (ax^2 + bx + c) to transform it into a perfect square trinomial. Here’s a breakdown of the steps:
- Identify the Coefficient of the x^2 Term (a): Notice the coefficient multiplying the x^2 term. This value is crucial for the following steps.
- Half the Coefficient (b/2): Divide the coefficient of the x term (b) by 2.
- Squaring the Result: Take the result from step 2 (b/2) and square it. This value represents the constant term we’ll add later.
- Reorganizing the Expression: Here’s the magic! We can manipulate the expression strategically. Split the bx term into two identical parts, add the result of step 3 (b^2 / 4) to both sides of the equation.
The logic behind this manipulation: We’re essentially “borrowing” from the expression and “paying it back” later in a way that allows us to create a perfect square trinomial.
- Creating the Perfect Square Trinomial: By following step 4, you’ll end up with a term that resembles (x + b/2)^2. This creates the perfect square trinomial on the left side of the equation.
Remember: Since we “borrowed” and “paid back” with the constant term, the overall value of the expression remains unchanged.
Examples in Action: Putting Theory into Practice
Let’s see how completing the square works with some practical examples:
Example 1: Factoring a Quadratic Expression
Factor the expression: x^2 + 6x + 5
-
Identify a (coefficient of x^2): In this case, a = 1.
-
Half the coefficient (b/2): b = 6, so b/2 = 3.
-
Square the result: (3)^2 = 9.
-
Reorganize and add the result of step 3 to both sides: (x^2 + 6x) + 5 = (x^2 + 6x + 9) + 5 – 9 (x^2 + 6x) + 5 = (x + 3)^2 – 4
-
Notice how the left side becomes a perfect square trinomial, allowing you to factor the expression: (x + 3)^2 – 4 = (x + 3 + 2)(x + 3 – 2) = (x + 5)(x + 1)
Example 2: Solving a Quadratic Equation
Solve the equation: x^2 – 4x + 7 = 0
-
Follow steps 1-3 from the previous example (a = 1, b = -4, b/2 = -2, (b/2)^2 = 4).
-
Reorganize and add the result of step 3 to both sides: x^2 – 4x + 7 = (x^2 – 4x + 4) + 7 – 4 x^2 – 4x + 7 = (x – 2)^2 + 3
-
Isolate the x term to solve for the roots.
Beyond the Basics: Exploring Advanced Applications of Completing the Square
While the core concept of completing the square focuses on manipulating quadratic expressions, its applications extend beyond basic factoring and solving equations. Here, we’ll delve into some advanced scenarios where completing the square proves its worth.
The Vertex Form and Understanding the Graph
Completing the square sheds light on the vertex form of a quadratic equation, which is written as f(x) = a(x – h)^2 + k. Here’s how it helps:
- Completing the square transforms the quadratic expression into a form resembling the vertex form.
- By analyzing the vertex form (a(x – h)^2 + k), we can identify the vertex’s coordinates (h, k). The vertex represents the point where the parabola reaches its minimum (for positive a) or maximum (for negative a).
This understanding empowers you to visualize the graph of the quadratic equation and predict its behavior.
The Quadratic Formula Revisited: A Different Perspective
The quadratic formula, a universal tool for solving quadratic equations, can be derived by completing the square. Here’s a glimpse into the connection:
- By completing the square, we manipulate the equation to isolate the variable term (x).
- Through this manipulation, the quadratic formula emerges as a mathematical expression revealing the solutions (roots) of the equation.
Understanding this connection provides a deeper appreciation for the concept of completing the square and its role in solving quadratic problems.
Complex Numbers and Completing the Square
While the focus of this guide has been on real numbers, completing the square can also be applied to quadratic expressions with complex number coefficients.
In these scenarios, the steps remain similar, but the resulting solutions might involve imaginary unit multiples (represented by the symbol ‘i’).
Understanding how to complete the square in these situations lays the groundwork for exploring more advanced mathematical concepts.
Beyond Equations: Completing the Square in Inequalities
The power of completing the square extends to quadratic inequalities as well. By manipulating the expression to isolate the variable term, you can analyze the inequality and determine the solution set (the range of x values that satisfy the inequality).
This application demonstrates the versatility of completing the square and its usefulness in various mathematical contexts.
Frequently Asked Questions: How to Complete the Square?
Q: What is the purpose of completing the square?
A: Completing the square helps factor quadratic expressions, solve quadratic equations, understand the vertex form of parabolas, and solve quadratic inequalities.
Q: What are the steps to complete the square?
A: The steps involve identifying the coefficient of the x^2 term, dividing the coefficient of the x term by 2 and squaring it, manipulating the expression strategically to create a perfect square trinomial, and factoring or solving for the variable.
Q: Can completing the square be used with complex numbers?
A: Yes, the core steps remain similar, but the solutions might involve imaginary unit multiples.