Understanding Polygons: Convex and Concave Shapes
Introduction
Polygons are two-dimensional shapes made up of straight lines that connect to form a closed figure. The classification of polygons can be based on various criteria, including the number of sides, the arrangement of angles, and whether they are convex or concave. This article will explore the characteristics of convex and concave polygons, focusing specifically on heptagons (seven-sided polygons) and hexagons (six-sided polygons).
What is a Polygon?
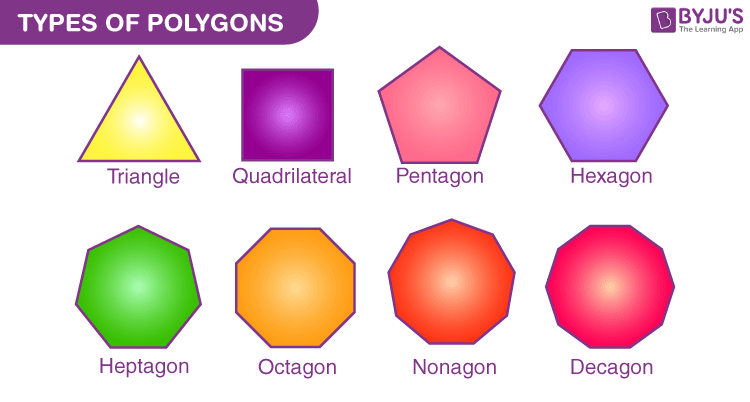
A polygon is defined as a closed figure formed by three or more straight line segments, called sides. The points where two sides meet are called vertices. Polygons can be classified based on the number of sides:
- Triangle: 3 sides
- Quadrilateral: 4 sides
- Pentagon: 5 sides
- Hexagon: 6 sides
- Heptagon: 7 sides
- Octagon: 8 sides
- Nonagon: 9 sides
- Decagon: 10 sides
Types of Polygons
Polygons can be categorized into two main types: convex and concave.
Convex Polygons
A convex polygon is defined as a polygon in which all interior angles are less than 180 degrees, and no line segment between any two points on the boundary ever goes outside the polygon. In simpler terms, if you were to draw a line between any two points inside the polygon, the line would remain inside the polygon.Characteristics of Convex Polygons:
- All interior angles are less than 180 degrees.
- No indentations or inward curves.
- The line segment connecting any two points within the polygon lies entirely inside the polygon.
Examples of Convex Polygons:
- Convex Hexagon
- Convex Heptagon
Concave Polygons
A concave polygon is defined as a polygon that has at least one interior angle greater than 180 degrees. This means that at least one vertex of the polygon points inward, creating an indentation.Characteristics of Concave Polygons:
- At least one interior angle is greater than 180 degrees.
- At least one line segment connecting two points within the polygon lies outside the polygon.
Examples of Concave Polygons:
- Concave Hexagon
- Concave Heptagon
Detailed Examination of Heptagons and Hexagons
Heptagons
A heptagon is a polygon with seven sides and seven vertices. Heptagons can be either convex or concave.
- Convex Heptagon
- All interior angles are less than 180 degrees.
- Example: A regular heptagon where all sides and angles are equal.
- Concave Heptagon
- At least one interior angle is greater than 180 degrees.
- Example: A heptagon with one vertex that points inward.
Hexagons
A hexagon is a polygon with six sides and six vertices. Like heptagons, hexagons can also be convex or concave.
- Convex Hexagon
- All interior angles are less than 180 degrees.
- Example: A regular hexagon where all sides and angles are equal.
- Concave Hexagon
- At least one interior angle is greater than 180 degrees.
- Example: A hexagon with one vertex that points inward.
Table of Polygon Characteristics
| Polygon Type | Number of Sides | Convex/Concave | Interior Angles Characteristics |
|---|---|---|---|
| Convex Heptagon | 7 | Convex | All angles < 180 degrees |
| Concave Heptagon | 7 | Concave | At least one angle > 180 degrees |
| Convex Hexagon | 6 | Convex | All angles < 180 degrees |
| Concave Hexagon | 6 | Concave | At least one angle > 180 degrees |
Visual Representation
To better understand the differences between these polygon types, consider the following visual representations:
- Convex Heptagon: A regular heptagon with equal sides and angles, resembling a star-like shape when drawn with equal angles.
- Concave Heptagon: A heptagon with one inward-pointing vertex, creating a “dent” in the shape.
- Convex Hexagon: A regular hexagon with equal sides and angles, often resembling a honeycomb structure.
- Concave Hexagon: A hexagon with one inward-pointing vertex, creating a similar “dent” in the shape.
Applications of Polygons
Polygons, both convex and concave, are widely used in various fields, including:
- Architecture: Designing buildings and structures often involves the use of polygons to create visually appealing shapes.
- Computer Graphics: Polygons are fundamental in 3D modeling and animation, where complex shapes are represented using simpler polygonal forms.
- Mathematics: Understanding the properties of polygons is essential in geometry, where they are studied for their angles, sides, and symmetry.
FAQ Section
Q1: What is the difference between a convex and a concave polygon?
A1: A convex polygon has all interior angles less than 180 degrees, while a concave polygon has at least one interior angle greater than 180 degrees.
Q2: How many sides does a heptagon have?
A2: A heptagon has seven sides.
Q3: Can a polygon be both convex and concave?
A3: No, a polygon cannot be both convex and concave. It is classified as one or the other based on its angles.
Q4: What is a regular polygon?
A4: A regular polygon is a polygon with all sides and angles equal. For example, a regular hexagon has six equal sides and angles.
Q5: What are some examples of concave polygons?
A5: Examples of concave polygons include concave heptagons and concave hexagons, which have at least one inward-pointing vertex.
Q6: Why are polygons important in mathematics?
A6: Polygons are fundamental shapes in geometry, and understanding their properties helps in various mathematical applications, including area and perimeter calculations.
Q7: How are polygons used in real life?
A7: Polygons are used in architecture, computer graphics, and various design fields to create shapes and structures.
Q8: What is the sum of the interior angles of a heptagon?
A8: The sum of the interior angles of a heptagon can be calculated using the formula (n−2)×180, where n is the number of sides. For a heptagon, it is (7−2)×180=900 degrees.
Q9: Can polygons have curved sides?
A9: No, by definition, polygons have straight sides. Shapes with curved sides are not classified as polygons.
Q10: Where can I find more information about polygons?
A10: For more detailed information about polygons, you can refer to the Wikipedia page on polygons.
Conclusion
Understanding the differences between convex and concave polygons, particularly heptagons and hexagons, is essential in geometry and various practical applications. By recognizing the characteristics of these shapes, one can appreciate their significance in mathematics, architecture, and design. Whether you are studying for academic purposes or simply exploring the world of shapes, the knowledge of polygons will enhance your understanding of geometry.